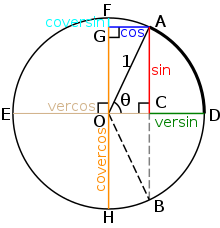
正矢

正矢函数（versine^{[2][3][4][5][6]}或versed sine^{[7][8][9][10][11]}）是一个三角函数，常计为versin(θ)、 sinver(θ)、^[12][13]vers(θ)、 ver(θ)^[14]或 siv(θ)。^[15][16] 在拉丁语中，其被称为sinus versus (翻转的正弦), versinus、 versus或 sagitta (箭头)。^[17]

其等价定义为

${\displaystyle \operatorname {versin} (\theta )=1-\cos(\theta )=2\sin ^{2}\left({\frac {\theta }{2}}\right)}$ 

相关函数

• 余的正矢（英文：versed cosine、vercosine）^[18]，写为vercosin(θ)、 vercos(θ)或 vcs(θ)。
• 余矢（英文：coversed sine、coversine）^[19]，写为${\displaystyle \operatorname {coversin} (\theta )}$ ，有时亦缩写为${\displaystyle \operatorname {cvs} (\theta )}$ 。
• 余的余矢（英文：coversed cosine^[20]或 covercosine），写为covercosin(θ)、 covercos(θ)或 cvc(θ)。

与上述四个函数类似，还存在四个“半值”函数：

• 半正矢（英文：haversed sine,^[21] haversine或 semiversus^[22][23]），写为haversin(θ)、 semiversin(θ)、 semiversinus(θ)、 havers(θ)、 hav(θ)、^[24][25] hvs(θ)、^{[注 1]} sem(θ)或 hv(θ)^[26]，因半正矢公式出名，且曾用于导航术。
• 余的半正矢（英文：haversed cosine^[27] or havercosine），写为havercosin(θ), havercos(θ), hac(θ)或 hvc(θ)。
• 半余矢（英文：hacoversed sine、hacoversine^[28]或 cohaversine），写为hacoversin(θ)、 semicoversin(θ)、 hacovers(θ)、 hacov(θ)^[29]或 hcv(θ)。
• 余的半余矢（英文：hacoversed cosine^[30]、 hacovercosine或 cohavercosine），写为hacovercosin(θ)、 hacovercos(θ)或 hcc(θ)。

• 三角函数

• Sagitta, Apothem, and Chord （页面存档备份，存于互联网档案馆） by Ed Pegg, Jr., The Wolfram Demonstrations Project.