梅滕斯猜想

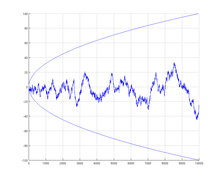
梅滕斯猜想是数论中的一个猜想，是有关数论中梅滕斯函数上下界的猜想，由汤姆斯·斯蒂尔吉斯在一封于1885年写给夏尔·埃尔米特与弗朗茨·梅滕斯（Franz Mertens）的信中提出。这一猜想如果成立的话可以推出黎曼猜想，不过已被安德鲁·奥德里兹科（英语：Andrew Odlyzko）与赫尔曼·特里尔（英语：Herman te Riele）于1985年证否。

图示为梅滕斯函数的前10000项与默滕斯猜想中的界限

\pm {\sqrt {n}}

。梅滕斯在计算梅滕斯函数的前一万个值之后，猜想

M(n)

的绝对值恒小于

{\sqrt {n}}

，此猜想被安德鲁·奥德里兹科（Andrew Odlyzko）与赫尔曼·特里尔（Herman te Riele）于1985年证否

定义

数论中，有梅滕斯函数

M(n)=\sum _{1\leq k\leq n}\mu (k)

其中， $\mu (k)$ 表示默比乌斯函数。则梅滕斯猜想是指，对所有 $n>1$ ，有

\left|M(n)\right|<{\sqrt {n}}.\,

猜想的证否

汤姆斯·斯蒂尔吉斯在1885年声称已证明比梅滕斯猜想要弱的结果，也就是 $m(n)\equiv M(n)/{\sqrt {n}}$ 有界，但其结果没有发表^[1]（若用 $m(n)$ 的方式表示，梅滕斯猜想是指 $-1<m(n)<1$ ）

安德鲁·奥德里兹科（英语：Andrew Odlyzko）与赫尔曼·特里尔（英语：Herman te Riele）在1985年证否了梅滕斯猜想，用的是LLL格缩减算法（英语：Lenstra–Lenstra–Lovász lattice basis reduction algorithm）^[2]^[3]：

\liminf ~m(n)<-1.009\quad

and

\quad \limsup ~m(n)>1.06~

之后也证实了第一个反例小于 $~e^{3.21\times 10^{64}}\approx 10^{1.39\times 10^{64}}~$ ^[4]，大于10¹⁶^[5]，后来的上限已降到 $~e^{1.59\times 10^{40}}~$ ^[6]或近似 $~10^{6.91\times 10^{39}}~,$ ，但还没找到确切的反例数值。

参考资料

^ Borwein, Peter; Choi, Stephen; Rooney, Brendan; Weirathmueller, Andrea (编). The Riemann hypothesis. A resource for the aficionado and virtuoso alike. CMS Books in Mathematics. New York, NY: 施普林格科学+商业媒体. 2007: 69. ISBN 978-0-387-72125-5. Zbl 1132.11047.
^ Odlyzko & te Riele (1985)
^ Sandor et al (2006) pp.188–189
^ Pintz (1987)
^ Hurst, Greg. Computations of the Mertens function and improved bounds on the Mertens conjecture. 2016. arXiv:1610.08551  [math.NT].
^ Kotnik and Te Riele (2006)

参考文献

T. Kotnik and Herman te Riele (2006), "The Mertens Conjecture Revisited^{[永久失效链接]}", Lecture Notes in Computer Science 4076 (Proceedings of the 7th Algorithmic Number Theory Symposium), pp. 156-167.
T. Kotnik and J. van de Lune (2004), "On the order of the Mertens function", Experimental Mathematics 13, pp. 473-481
F. Mertens (1897), "Über eine zahlentheoretische Funktion", Sitzungsberichte der Kaiserlichen Akademie der Wissenschaften, Mathematisch-Naturwissenschaftliche Klasse, Abteilung 2a, 106, pp. 761-830.
Odlyzko, A. M.; te Riele, H. J. J., Disproof of the Mertens conjecture (PDF), Journal für die reine und angewandte Mathematik, 1985, 357: 138–160 [2012-09-08], ISSN 0075-4102, doi:10.1515/crll.1985.357.138, MR783538, （原始内容存档 (PDF)于2015-09-12）
Stieltjes, T. J., Lettre a Hermite de 11 juillet 1885, Lettre #79, Baillaud, B.; Bourget, H. (编), Correspondance d’Hermite et Stieltjes, Paris: Gauthier—Villars: 160–164, 1905
埃里克·韦斯坦因. Mertens conjecture. MathWorld.
Sándor, József; Mitrinović, Dragoslav S.; Crstici, Borislav (编), Handbook of number theory I, Dordrecht: Springer-Verlag: 187–189, 2006, ISBN 1-4020-4215-9, Zbl 1151.11300
Pintz, J. An effective disproof of the Mertens conjecture (PDF). Astérisque. 1987,. 147–148: 325–333 [2021-10-16]. Zbl 0623.10031. （原始内容存档 (PDF)于2021-04-15）.

[1] Borwein, Peter; Choi, Stephen; Rooney, Brendan; Weirathmueller, Andrea (编). The Riemann hypothesis. A resource for the aficionado and virtuoso alike. CMS Books in Mathematics. New York, NY: 施普林格科学+商业媒体. 2007: 69. ISBN 978-0-387-72125-5. Zbl 1132.11047.

[2] Odlyzko & te Riele (1985)

[HBI1889-3] Sandor et al (2006) pp.188–189

[4] Pintz (1987)

[5] Hurst, Greg. Computations of the Mertens function and improved bounds on the Mertens conjecture. 2016. arXiv:1610.08551  [math.NT].

[6] Kotnik and Te Riele (2006)

[1]

[2]

[3]

[4]

[5]

[6]