小三角六边形二十面体
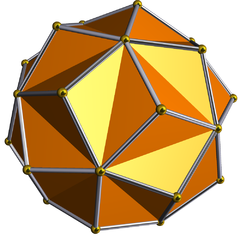
在几何学中,小三角六边形二十面体是一种星形二十面体[1][2],由20个等边但不等角且互相相交的六边形组成[3],其索引编号为DU30。温尼尔在他的书中列出28种星形多面体模型,并将小三角六边形二十面体给予编号W26[4]。其也收录于哈罗德·斯科特·麦克唐纳·考克斯特的书《五十九种二十面体》中,编号为2[5]。
 | ||
| 类别 | 星形多面体 | |
|---|---|---|
| 对偶多面体 | 小双三斜三十二面体 | |
| 识别 | ||
| 名称 | 小三角六边形二十面体 | |
| 参考索引 | DU30, 2/59, W26 | |
| 性质 | ||
| 面 | 20 | |
| 边 | 60 | |
| 顶点 | 32 | |
| 欧拉特征数 | F=20, E=60, V=32 (χ=-8) | |
| 亏格 | 5 | |
| 组成与布局 | ||
| 面的种类 | 20个等边六边形 | |
| 顶点布局 | 两种顶点 5个六边形的公共顶点 3个六边形的公共顶点 | |
| 对称性 | ||
| 对称群 | Ih, H3, [5,3], (*532) | |
| 特性 | ||
| 等面、等边 | ||
| 图像 | ||
| ||
小三角六边形二十面体的对偶多面体是一种均匀多面体,是由五角星和三角形组成的小双三斜三十二面体。
性质
小三角六边形二十面体由20个面、60条边和32个顶点组成[6][7],每个面都是等边六边形,但不是正六边形,每个六边形彼此互相相交,其共存在两种顶角,分别为5个六边形的公共顶点和3个六边形的公共顶点
作为星形多面体
作为一个星形多面体,其具有正二十面体的星状核和五角化十二面体的凸包。
| 星状图 | 外观 | 星状核 | 凸包 |
|---|---|---|---|
| 正二十面体 |
五角化十二面体 |
作为凹多面体
若作为凹多面体,即将原本互相相交的六边形面去除隐没部分其余部分分为3个三角形,这种结构与星形三角化二十面体相同
| 星形三角化二十面体 | 小三角六边形二十面体 | 三角化二十面体 | 正二十面体 |
|---|---|---|---|
顶点坐标
小三角六边形二十面体的顶点坐标为[8]:
二面角
体积与表面积
一个边长为a的小三角六边形二十面体,其表面积和体积为[10]:
其中A代表表面积 约为11倍的边长平方、V代表体积 约为3倍的边长立方。
相关多面体
对偶复合体
小三角六边形二十面体与其对偶的复合体为复合小双三斜三十二面体小三角六边形二十面体。其共有52个面、120条边和52个顶点,其尤拉示性数为-16,亏格为9,有12个非凸面,在威佐夫记号中以(3 | 5/2 3)表示[11]。
参考文献
- Wenninger, Magnus. Polyhedron Models. Cambridge University Press. 1974. ISBN 0-521-09859-9.
- Wenninger, Magnus. Dual Models. Cambridge University Press. 1983. ISBN 0-521-54325-8.
- Coxeter, Harold Scott MacDonald; Du Val, P.; Flather, H. T.; Petrie, J. F. The fifty-nine icosahedra 3rd. Tarquin. 1999. ISBN 978-1-899618-32-3. MR676126. (1st Edn University of Toronto (1938))
- ^ Topological Small Triambic Icosahedron. software3d.com. [2016-09-01]. (原始内容存档于2015-09-21).
- ^ Maeder, R. E. "The Stellated Icosahedra." (页面存档备份,存于互联网档案馆) Mathematica in Education 3, 5-11, 1994.
- ^ Grünbaum, Branko. Can Every Face of a Polyhedron Have Many Sides? (PDF). digital.lib.washington.edu. 2008 [2021-07-19]. (原始内容存档 (PDF)于2021-07-19).
- ^ Wenninger, Magnus. Polyhedron Models. Cambridge University Press. 1974. ISBN 0-521-09859-9.
- ^ H·S·M·考克斯特. 五十九種二十面體. H. T. Flather, J. F. Petrie. Springer Science & Business Media. 2012. ISBN 9781461382164.
- ^ small triambic icosahedron. bulatov.org. [2016-09-01]. (原始内容存档于2015-09-06).
- ^ Small triambic icosahedron 3D model. craftsmanspace. [2016-09-01]. (原始内容存档于2016-08-10).
- ^ Data of Small Triambic Icosahedron. dmccooey.com. [2016-09-01]. (原始内容存档于2016-09-01).
- ^ Versi-Regular Polyhedra: Small Triambic Icosahedron. dmccooey.com. [2016-09-01]. (原始内容存档于2016-03-24).
- ^ Weisstein, Eric W. (编). Small Triambic Icosahedron. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语). (原始 (页面存档备份,存于互联网档案馆)内容于2016-08-01).
- ^ compound of small ditrigonal icosidodecahedron and small triambic icosahedron. bulatov.org. [2016-09-01]. (原始内容存档于2015-09-06).
外部链接
- 埃里克·韦斯坦因. 小三角六邊形二十面體. MathWorld.
- 如何制作小三角六边形二十面体 (页面存档备份,存于互联网档案馆) crafts4camp.com (英文)
