余弦定理

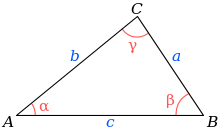
余弦定理的历史可追溯至公元三世纪前欧几里得的几何原本，在书中将三角形分为钝角和锐角来解释，这同时对应现代数学中余弦值的正负。根据几何原本第二卷的命题12和13^[1]，并参考右图，以现代的数学式表示即为：

${\displaystyle {\overline {AB}}^{2}={\overline {CA}}^{2}+{\overline {CB}}^{2}+2({\overline {CA}})({\overline {CH}})\,}$ 

其中${\displaystyle {\overline {CH}}={\overline {BC}}\cos(\pi -\gamma )=-{\overline {BC}}\cos \gamma }$ ，将其带入上式得到：

${\displaystyle {\overline {AB}}^{2}={\overline {CA}}^{2}+{\overline {CB}}^{2}-2({\overline {CA}})({\overline {BC}})\cos \gamma }$ 

三角函数

见右图，在${\displaystyle c}$ 上做高可以得到（投影定理）：

${\displaystyle c=a\cos \beta +b\cos \alpha }$ 

将等式同乘以c得到：

${\displaystyle c^{2}=ac\cos \beta +bc\cos \alpha }$ 

运用同样的方式可以得到：

${\displaystyle a^{2}=ac\cos \beta +ab\cos \gamma }$ 

${\displaystyle b^{2}=bc\cos \alpha +ab\cos \gamma }$ 

将${\displaystyle c^{2}}$ 的右式取代：

${\displaystyle c^{2}=ac\cos \beta +bc\cos \alpha =(a^{2}-ab\cos \gamma )+(b^{2}-ab\cos \gamma )=a^{2}+b^{2}-2ab\cos \gamma }$ 

勾股定理

勾股定理之一

设${\displaystyle \triangle ABC}$ 中，${\displaystyle {\overline {AB}}=c}$ ，${\displaystyle {\overline {BC}}=a}$ ，${\displaystyle {\overline {AC}}=b}$ 。过${\displaystyle B}$ 点作${\displaystyle AC}$ 的垂线，垂足为${\displaystyle D}$ ，如果${\displaystyle D}$ 在${\displaystyle AC}$ 内部，则${\displaystyle BD}$ 的长度为${\displaystyle a\sin C}$ ，${\displaystyle DC}$ 的长度为${\displaystyle a\cos C}$ ，${\displaystyle AD}$ 的长度为${\displaystyle b-a\cos C}$ 。根据勾股定理：

${\displaystyle c^{2}=(a\sin C)^{2}+(b-a\cos C)^{2}\,}$ 

${\displaystyle c^{2}=a^{2}\sin ^{2}C+b^{2}-2ab\cos C+a^{2}\cos ^{2}C\,}$ 

${\displaystyle c^{2}=a^{2}(\sin ^{2}C+\cos ^{2}C)+b^{2}-2ab\cos C\,}$ 

${\displaystyle c^{2}=a^{2}+b^{2}-2ab\cos C\,}$ 

如果${\displaystyle D}$ 在${\displaystyle AC}$ 的延长线上，证明是类似的。同理可以得到其他的等式。

勾股定理之二

设${\displaystyle \triangle ABC}$ 中，${\displaystyle {\overline {AB}}=c}$ ，${\displaystyle {\overline {BC}}=a}$ ，${\displaystyle {\overline {AC}}=b}$ 。过${\displaystyle B}$ 点作${\displaystyle {\overline {AC}}}$ 的垂线，垂足为${\displaystyle D}$ ，设${\displaystyle {\overline {AD}}=x}$ ，则${\displaystyle {\overline {CD}}=b-x}$ ，根据勾股定理：

${\displaystyle c^{2}-x^{2}={\overline {BD}}^{2}=a^{2}-(b-x)^{2}}$ 

${\displaystyle c^{2}-x^{2}=a^{2}-b^{2}-x^{2}+2bx}$ 

${\displaystyle c^{2}=a^{2}-b^{2}+2bx}$ 

${\displaystyle x={\frac {b^{2}+c^{2}-a^{2}}{2b}}}$ 

${\displaystyle \cos A={\frac {x}{c}}={\frac {b^{2}+c^{2}-a^{2}}{2bc}}}$ 

如果${\displaystyle D}$ 在${\displaystyle {\overline {AC}}}$ 的延长线上，证明是类似的。同理可以得到其他的等式。

余弦定理是解三角形中的一个重要定理。

求边

余弦定理可以简单地变形成：

${\displaystyle a={\sqrt {b^{2}+c^{2}-2bc\cos A}}}$ 

${\displaystyle b={\sqrt {c^{2}+a^{2}-2ac\cos B}}}$ 

${\displaystyle c={\sqrt {a^{2}+b^{2}-2ab\cos C}}}$ 

因此，如果知道了三角形的两边及其夹角，可由余弦定理得出已知角的对边。

求角

余弦定理可以简单地变形成：

${\displaystyle \cos A={\frac {b^{2}+c^{2}-a^{2}}{2bc}}\,\!}$ 

${\displaystyle \cos B={\frac {c^{2}+a^{2}-b^{2}}{2ca}}\,\!}$ 

${\displaystyle \cos C={\frac {a^{2}+b^{2}-c^{2}}{2ab}}\,\!}$ 

因为余弦函数在${\displaystyle ({{\rm {0}},\pi })}$ 上的单调性，可以得到：

${\displaystyle \angle A=\arccos {\frac {b^{2}+c^{2}-a^{2}}{2bc}}\,\!}$ 

${\displaystyle \angle B=\arccos {\frac {c^{2}+a^{2}-b^{2}}{2ca}}\,\!}$ 

${\displaystyle \angle C=\arccos {\frac {a^{2}+b^{2}-c^{2}}{2ab}}\,\!}$ 

因此，如果已知三角形的三边，可以由余弦定理得到三角形的三个内角。

• 三角形
• 勾股定理
• 正弦定理
• 正切定理
• 角平分线长公式
• 中线长公式

1. ^ In obtuse-angled triangles the square on the side subtending the obtuse angle is greater than the squares on the sides containing the obtuse angle by twice the rectangle contained by one of the sides about the obtuse angle, namely that on which the perpendicular falls, and the straight line cut off outside by the perpendicular towards the obtuse angle. --- Euclid's Elements, translation by Thomas L. Heath.