正图形列表
| 正多边形(二维) | |
|---|---|
| 凸 | 星形 |
 {5} |
 {5/2} |
| 正多面体(三维) | |
| 凸 | 星形 |
 {5,3} |
 {5/2,5} |
| 正镶嵌图(二维) | |
| 平面 | 双曲 |
 {4,4} |
 {5,4} |
| 正多胞体(四维) | |
| 凸 | 星形 |
 {5,3,3} |
 {5/2,5,3} |
| 正堆砌体(三维) | |
| 平面 | 双曲 |
 {4,3,4} |
 {5,3,4} |
此页面列出了所有的欧几里得空间、双曲空间和球形空间的正图形或正多胞形。施莱夫利符号可以描述每一个正图形或正多胞形,他被广泛使用如下面的每一个紧凑的参考名称。
正图形或正多胞形可由其维度分类,也可以分成凸、非凸(星形、扭歪、复合或凹)和无穷等形式。非凸形式(或凹形式)使用与凸形式相同的顶点,但面(或边)有相交。无限的形式则是在一较低维的欧几里得空间中密铺(镶嵌或堆砌)。
无限的形式可以扩展到密铺双曲空间。双曲空间是和正常的空间有相同的规模,但平行线在一定的距离内会分岔得越来越远。这使得顶点值可以存在负角度的缺陷,例如制作一个由个正三角形组成的顶点,它们可以被平放。它不能在普通平面上完成的,但可以在一个双曲平面上构造。
概观
此表显示正图形或正多胞形在各个维度的汇总。
| 有限[注 1] | 平面[注 2] | 双曲[注 3] | 复合[注 4] | 抽象 | ||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 维度 | 凸 | 非凸 | 密铺 | |||||||||||||||
| 星形 | 扭歪 | 凸 | 星形 | 扭歪 | 紧凑 | 星形 | 仿紧 | 非紧 | 凸 | 星形 | ||||||||
| -1[注 5] | 0 | 0 | 0 | 0 | 0[注 6] | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1[注 7] | |||||
| 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | ||||||
| 1 | 1 | 0 | 0 | 1[注 8] | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | ||||||
| 2 | ∞ | ∞ | ∞ | 1 | 1 | 1 | 0 | 0 | 0 | ∞ | ∞ | ∞ | ||||||
| 3 | 5 | 4 | ? | 3 | 3 | ∞ | ∞ | ∞ | ∞ | 5 | 0 | ∞ | ||||||
| 4 | 6 | 10 | ? | 1 | ? | 4 | 0 | 11 | ∞ | 26 | 20 | ∞ | ||||||
| 5 | 3 | 0 | ? | 3 | ? | 5 | 4 | 2 | 186[1] | 0 | 0 | ∞ | ||||||
| 6 | 3 | 0 | ? | 1 | ? | 0 | 0 | 5 | 66[1] | 0 | 0 | ∞ | ||||||
| 7 | 3 | 0 | ? | 1 | ? | 0 | 0 | 0 | 36[1] | 3 | 0 | ∞ | ||||||
| 8 | 3 | 0 | ? | 1 | ? | 0 | 0 | 0 | 13[1] | 6 | 0 | ∞ | ||||||
| 9 | 3 | 0 | ? | 1 | ? | 0 | 0 | 0 | 10[1] | 0 | 0 | ∞ | ||||||
| 10 | 3 | 0 | ? | 1 | ? | 0 | 0 | 0 | 8[1] | 0 | 0 | ∞ | ||||||
| 11 | 3 | 0 | ? | 1 | ? | 0 | 0 | 0 | 4[1] | 0 | 0 | ∞ | ||||||
| 12+ | 3 | 0 | ? | 1 | ? | 0 | 0 | 0 | [2] | ≤2[注 9] | 0 | ∞ | ||||||
| ||||||||||||||||||
零维或以下的正图形
在维数为零的空间能存在的多胞形只有点[3],无法有其他几何或拓朴组合,而维数比零更低则是在抽象理论中的虚无多胞形(英语:Null polytope)代表一种空集合,在抽象理论中被看作是一种负一维的多胞形[4],但其是一种抽象多胞形。然而,在数学上,零维空间是按以下的不等价定义之一,维数为零的拓扑空间:按覆盖维数的概念,一个拓扑空间是零维空间,若空间的任何开覆盖,都有一个加细,使得空间内每一点,都在这个加细的恰好一个开集内;或者按小归纳维数的概念,一个拓扑空间是零维空间,若空间有一个由闭开集组成的基。这两个概念对可分可度量化空间为等价[5][6]。而负一维空间仅是在抽象理论表示一个比零维多胞形更低维度的一个元词。
依据正图形的定义,一个多胞形必须要具备严格的标记可递特性,对于该几何体内所有同维度的元素(如:点、线、面)都完全具有相同的性质,并且每一个元素皆为一个正图形,而零维多胞形的元素仅有{F−1, F0}、负一维多胞形的元素仅有{F−1},几何上所有零维多胞形都是正多胞形,一般地,n维正图形被定义为有正维面[(n − 1)-表面]和正顶点图,这两个条件已经能充分地保证所有面、所有顶点都是相似的,但这一定义并不适用于抽象多胞形,而负一维的多胞形的仅有一种抽象多胞形。
另外,正零边形也可以视为零维或以下的正图形,或看做是虚无多胞形(英语:Null polytope)。
一维正图形

|
考克斯特记号终结点代表一个镜射面,周围有环的节点表示其不位于一个平面。 ditel, { }, |
在维数为一的一维空间里存在的多胞形是由两个端点包围住的一个封闭一维空间,即线段。在定义上,这个一维多胞形(或称1-多胞形)在施莱夫利符号中以: { } 表示[8][9],而在考克斯特记号中则以一个有环的节点:![]() 表示[7]。诺曼·约翰逊将之称为ditel,并在施莱夫利符号中以{ }表示[10]。依据正图形的定义,一个多胞形必须要具备严格的标记可递特性,对于该几何体内所有同维度的元素(如:点、线、面)都完全具有相同的性质,并且每一个元素皆为一个正图形,而一维多胞形的旗包含{F−1, F0, F1}、其元素仅有{F−1, A, B, AB},其中,A、B为线段两端点,由于几何上所有零维多胞形都是正多胞形,因此所有的线段都会符合标记可递特以及所有同维度的元素(如:点、线、面)都完全具有相同的性质,并且每一个元素皆为一个正图形,因此在几何上所有的一维多胞形都是正多胞形。
表示[7]。诺曼·约翰逊将之称为ditel,并在施莱夫利符号中以{ }表示[10]。依据正图形的定义,一个多胞形必须要具备严格的标记可递特性,对于该几何体内所有同维度的元素(如:点、线、面)都完全具有相同的性质,并且每一个元素皆为一个正图形,而一维多胞形的旗包含{F−1, F0, F1}、其元素仅有{F−1, A, B, AB},其中,A、B为线段两端点,由于几何上所有零维多胞形都是正多胞形,因此所有的线段都会符合标记可递特以及所有同维度的元素(如:点、线、面)都完全具有相同的性质,并且每一个元素皆为一个正图形,因此在几何上所有的一维多胞形都是正多胞形。
虽然线段做为一个多胞形是微不足道的,但它似乎是多边形和其他更高维度图形形成边缘所需的一个元素[11]。在一维以及以下(包括一维、零维、负一维)空间中的多胞形都是正多胞形,包含了一维的线段、零维的点和负一维的抽象虚无多胞形都是组成多边形和其他更高维度图形的重要元素之一,比如一维的线段组成多边形的边、零维的点组成多边形的顶点以及代表集合子集中空集合的抽象虚无多胞形都是多边形的组成元素(子集),依据正图形定义,若这些低为度不存在正图形,则也不会有正多边形和其他更高维度的正图形。
在柱体的定义里,线段(一维)可以被看做是点(零维)的柱体,在施莱夫利符号中以{ }×{p}表示,而在考克斯特记号中则以笛卡儿积的形式![]()
![]()
![]()
![]()
![]() 表示一个线段和多边形[12]。
表示一个线段和多边形[12]。
二维正多边形
二维凸正多边形
| 名称 | 正三角形 (2-单体) |
正方形 (2-正轴形) (2-立方形) |
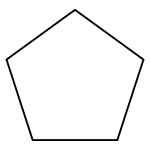
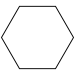
正五边形 | 正六边形 | 正七边形 | 正八边形 | |
|---|---|---|---|---|---|---|---|
| 施莱夫利符号 | {3} | {4} | {5} | {6} | {7} | {8} | |
| 考克斯特符号 | |||||||
| 图像 | 
|

|

|
|

|
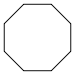
| |
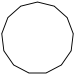
| 名称 | 正九边形 | 正十边形 | 正十一边形 | 正十二边形 | 正十三边形 | 正十四边形 | |
| 施莱夫利 | {9} | {10} | {11} | {12} | {13} | {14} | |
| 考克—迪肯 | |||||||
| 图像 | 
|

|

|

|
|

| |
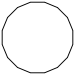
| 名称 | 正十五边形 | 正十六边形 | 正十七边形 | 正十八边形 | 正十九边形 | 正二十边形 | ...正n边形 |
| 施莱夫利 | {15} | {16} | {17} | {18} | {19} | {20} | {n} |
| 考克—迪肯 | |||||||
| 图像 | 
|
|

|

|

|

|
- 边数较大的正多边形
| 名称 | 二百五十七边形 | 正65537边形 | 一百万边形 |
|---|---|---|---|
| 可作图? | 可作图[13][14] | 可作图[15][16] | 不可 |
| 施莱夫利符号 | {257}[17] | {65537}[18] | {1000000}[19] |
| 考克斯特符号 | |||
| 图像 | 
|

|
 [20][注 10] [20][注 10]
|
退化 (圆形)
| 名称 | 正零边形 | 正一边形 | 正二边形 |
|---|---|---|---|
| 施莱夫利符号 | {1}[21] | {2}[22] | |
| 考克斯特符号 | |||
| 图像 | 
|

|
星形正多边形
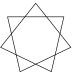
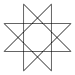
| 名称 | 五角星 | 七角星 | 八角星 | 九角星 | 十角星 | ...n角星 | ||
|---|---|---|---|---|---|---|---|---|
| 施莱夫利符号 | {5/2}[23] | {7/2} | {7/3} | {8/3} | {9/2} | {9/4} | {10/3} | {p/q} |
| 考克斯特符号 | ||||||||
| 图像 | 
|
|

|
|

|
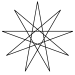
|

|
|
扭歪正多边形
| 扭歪六边形 | 扭歪八边形 | 扭歪十边形 | ||
| D3d, [2+,6] | D4d, [2+,8] | D5d, [2+,10] | ||
|---|---|---|---|---|
| {3}#{ } | {4}#{ } | {5}#{ } | {5/2}#{ } | {5/3}#{ } |

|
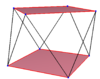
|

|

|
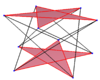
|
三维正图形
三维凸正多面体
| 名称 | 施莱夫利符号 {p,q} |
考克斯特符号 |
图像 (透视图) |
图像 (立体图) |
图像 (球面投影) |
面 {p} |
边 | 顶点 {q} |
对称群 | 对偶 |
|---|---|---|---|---|---|---|---|---|---|---|
| 正四面体 (3-单体) (三角锥) |
{3,3} | 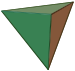
|

|

|
4 {3} |
6 | 4 {3} |
Td | (自身对偶) | |
| 正方体 (3-立方形) (正六面体) (四角柱) |
{4,3} | 
|

|

|
6 {4} |
12 | 8 {3} |
Oh | 正八面体 | |
| 正八面体 (3-正轴体) (正三角反棱柱) |
{3,4} | 
|

|

|
8 {3} |
12 | 6 {4} |
Oh | 立方体 | |
| 正十二面体 | {5,3} | 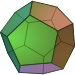
|

|

|
12 {5} |
30 | 20 {3} |
Ih | 正二十面体 | |
| 正二十面体 | {3,5} | 
|

|

|
20 {3} |
30 | 12 {5} |
Ih | 正十二面体 |
退化 (球面)
在球面几何学中,多面形 {2,n} 和多边形二面体 {n,2} 以及一面体 {1,1} 也可以被视为是一种正多面体(正球面镶嵌)。
他们包括:
| 名称 | 施莱夫利 {p,q} |
考克斯特 记号 |
图像 (球面) |
面 {p} |
边 | 顶点 {q} |
对称性 | 对偶 |
|---|---|---|---|---|---|---|---|---|
| 一边形一面体 | {1,1} | 
|
1 {1} |
0 | 1 {1} |
C1 (*1) |
自身对偶 | |
| 一边形二面体 | {1,2} | 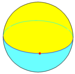
|
2 {1} |
1 | 1 {2} |
C1v (*22) |
一面形 | |
| 一面形 | {2,1} | 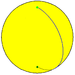
|
1 {2} |
1 | 2 {1} |
C1v (*22) |
一边形二面体 | |
| 二边形二面体 二面形 |
{2,2} | 
|
2 {2} |
2 | 2 {2} |
D2h (*222) |
自身对偶 | |
| 三面形 | {2,3} | 
|
3 {2} |
3 | 2 {3} |
D3h (*322) |
三角形二面体 | |
| 三角形二面体 | {3,2} | 
|
2 {3} |
3 | 3 {2} |
D3h (*322) |
三面形 | |
| 六面形 | {2,6} | 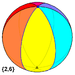
|
6 {2} |
6 | 2 {6} |
D6h (*622) |
六边形二面体 | |
| 六边形二面体 | {6,2} | 
|
2 {6} |
6 | 6 {2} |
D6h (*622) |
六面形 |
星形正多面体
| 名称 | 半透明 图像 |
立体 图像 |
球面镶嵌 图像 |
星状图 | 施莱夫利 {p,q} 考克斯特 |
面 {p} |
边 | 顶点 {q} 顶点图 |
χ | 密度 | 对称姓 | 对偶 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 小星形十二面体 | 
|

|
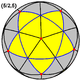
|

|
{5/2,5} |
12 {5/2} |
30 | 12 {5} |
−6 | 3 | Ih [5,3] (*532) |
大十二面体 |
| 大十二面体 | 
|

|

|

|
{5,5/2} |
12 {5} |
30 | 12 {5/2} |
−6 | 3 | Ih [5,3] (*532) |
小星形十二面体 |
| 大星形十二面体 | 
|

|

|

|
{5/2,3} |
12 {5/2} |
30 | 20 {3} |
2 | 7 | Ih [5,3] (*532) |
大二十面体 |
| 大二十面体 | 
|

|

|

|
{3,5/2} |
20 {3} |
30 | 12 {5/2} |
2 | 7 | Ih [5,3] (*532) |
大星形十二面体 |
扭歪正多面体
考克斯特在他的论文《三维和四维空间的正扭歪多面体极其类似物》[24]中列出了较多的一系列扭歪多面体,其中有四种是正图形。

|

|

|

|
| {4, 6 | 3} | {6, 4 | 3} | {4, 8 | 3} | {8, 4 | 3} |
|---|
四维正图形
四维凸正多胞体
在四维空间中存在6种凸正图形。
| 名称 |
施莱夫利 {p,q,r} |
考克斯特 |
胞 {p,q} |
面 {p} |
边 {r} |
顶点 {q,r} |
对偶 {r,q,p} |
|---|---|---|---|---|---|---|---|
| 正五胞体 (四维单纯形) |
{3,3,3} | 5 {3,3} |
10 {3} |
10 {3} |
5 {3,3} |
自身对偶 | |
| 正八胞体 (四维超方形) (超立方体) |
{4,3,3} | 8 {4,3} |
24 {4} |
32 {3} |
16 {3,3} |
正十六胞体 | |
| 正十六胞体 (四维正轴体) |
{3,3,4} | 16 {3,3} |
32 {3} |
24 {4} |
8 {3,4} |
超立方体 | |
| 正二十四胞体 | {3,4,3} | 24 {3,4} |
96 {3} |
96 {3} |
24 {4,3} |
自身对偶 | |
| 正一百二十胞体 (四维类五边形体) |
{5,3,3} | 120 {5,3} |
720 {5} |
1200 {3} |
600 {3,3} |
正六百胞体 | |
| 正六百胞体 (四维类二十面体体 |
{3,3,5} | 600 {3,3} |
1200 {3} |
720 {5} |
120 {3,5} |
正一百二十胞体 |
| 正五胞体 | 超立方体 | 正十六胞体 | 正二十四胞体 | 正一百二十胞体 | 正六百胞体 |
|---|---|---|---|---|---|
| {3,3,3} | {4,3,3} | {3,3,4} | {3,4,3} | {5,3,3} | {3,3,5} |
| 线架图 (皮特里多边形) 歪斜正投影图 | |||||

|
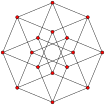
|

|
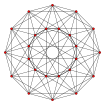
|

|
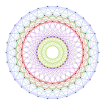
|
| 不透明正投影图 | |||||
 被正四面体包覆 (以顶点与胞为中心) |
 被立方体包覆 (以胞为中心) |
 被立方体包覆 (以胞为中心) |
 被截半立方体包覆 (以胞为中心) |
 被倒角十二面体包覆 (以胞为中心) |
 被五角化截半二十面体包覆 (以顶点为中心) |
| 施莱盖尔线框 (透视投影) | |||||
 (以胞为中心) |
 (以胞为中心) |
 (以胞为中心) |
 (以胞为中心) |
 (以胞为中心) |
 (以顶点为中心) |
| 球极平面投影线框 (超球面堆砌) | |||||

|
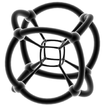
|

|

|

|

|
退化 (超球面)
| 施莱夫利 {2,p,q} |
考克斯特符号 |
胞 {2,p}π/q |
面 {2}π/p,π/q |
边 | 顶点 | 顶点图 {p,q} |
对称性 | 对偶多胞形 |
|---|---|---|---|---|---|---|---|---|
| {2,3,3} | 4 {2,3}π/3 
|
6 {2}π/3,π/3 |
4 | 2 | {3,3}
|
[2,3,3] | {3,3,2} | |
| {2,4,3} | 6 {2,4}π/3 |
12 {2}π/4,π/3 |
8 | 2 | {4,3}
|
[2,4,3] | {3,4,2} | |
| {2,3,4} | 8 {2,3}π/4 
|
12 {2}π/3,π/4 |
6 | 2 | {3,4}
|
[2,4,3] | {4,3,2} | |
| {2,5,3} | 12 {2,5}π/3 
|
30 {2}π/5,π/3 |
20 | 2 | {5,3}
|
[2,5,3] | {3,5,2} | |
| {2,3,5} | 20 {2,3}π/5 
|
30 {2}π/3,π/5 |
12 | 2 | {3,5}
|
[2,5,3] | {5,3,2} |
扭歪多胞体
四维的扭歪多胞体是一些位于五维或以上的扭歪图形。
五维正图形
五维凸正多胞体
| 名称 | 施莱夫利 {p,q,r,s} 考克斯特 |
维面 {p,q,r} |
胞 {p,q} |
面 {p} |
边 | 顶点 | 面图 {s} |
边图 {r,s} |
顶点图 {q,r,s} |
|---|---|---|---|---|---|---|---|---|---|
| 五维正六胞体 | {3,3,3,3} |
6 {3,3,3} |
15 {3,3} |
20 {3} |
15 | 6 | {3} | {3,3} | {3,3,3} |
| 五维超正方体 | {4,3,3,3} |
10 {4,3,3} |
40 {4,3} |
80 {4} |
80 | 32 | {3} | {3,3} | {3,3,3} |
| 五维正三十二胞体 | {3,3,3,4} |
32 {3,3,3} |
80 {3,3} |
80 {3} |
40 | 10 | {4} | {3,4} | {3,3,4} |
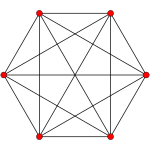 五维正六胞体 |
 五维超正方体 |
 五维正三十二胞体 |
六维正图形
六维凸正多胞体
| 名称 | 施莱夫利 | 顶点 | 边 | 面 | 胞 | 维脊 | 维面 | χ |
|---|---|---|---|---|---|---|---|---|
| 六维正七胞体 | {3,3,3,3,3} | 7 | 21 | 35 | 35 | 21 | 7 | 0 |
| 六维超立方体 | {4,3,3,3,3} | 64 | 192 | 240 | 160 | 60 | 12 | 0 |
| 六维正六十四胞体 | {3,3,3,3,4} | 12 | 60 | 160 | 240 | 192 | 64 | 0 |
 六维正七胞体 |
 六维超立方体 |
 六维正六十四胞体 |
七维正图形
七维凸正多胞体
| 名称 | 施莱夫利 | 顶点 | 边 | 面 | 胞 | 维峰 | 维脊 | 维面 | χ |
|---|---|---|---|---|---|---|---|---|---|
| 七维正八胞体 | {3,3,3,3,3,3} | 8 | 28 | 56 | 70 | 56 | 28 | 8 | 2 |
| 七维超立方体 | {4,3,3,3,3,3} | 128 | 448 | 672 | 560 | 280 | 84 | 14 | 2 |
| 七维正一百二十八胞体 | {3,3,3,3,3,4} | 14 | 84 | 280 | 560 | 672 | 448 | 128 | 2 |
 七维正八胞体 |
 七维超立方体 |
 七维正一百二十八胞体 |
七维以上正图形
n维凸正多胞体
从五维开始,凸正多胞体都只有三种[25]。
| 名称 | 施莱夫利 符号 {p1,...,pn−1} |
考克斯特 记号 |
k维胞 / 面 | 维面 | 顶点图 | 对偶 |
|---|---|---|---|---|---|---|
| n维单体 | {3n−1} | {3n−2} | {3n−2} | 自身对偶 | ||
| n维超立方体 | {4,3n−2} | {4,3n−3} | {3n−2} | n维正轴体 | ||
| n维正轴体 | {3n−2,4} | {3n−2} | {3n−3,4} | n维超立方体 |
八维
| 名称 | 施莱夫利 | 顶点 | 边 | 面 | 胞 | 4维胞 | 维峰 | 维脊 | 维面 | χ |
|---|---|---|---|---|---|---|---|---|---|---|
| 八维单体 | {3,3,3,3,3,3,3} | 9 | 36 | 84 | 126 | 126 | 84 | 36 | 9 | 0 |
| 八维超立方体 | {4,3,3,3,3,3,3} | 256 | 1024 | 1792 | 1792 | 1120 | 448 | 112 | 16 | 0 |
| 八维正轴体 | {3,3,3,3,3,3,4} | 16 | 112 | 448 | 1120 | 1792 | 1792 | 1024 | 256 | 0 |
 八维单体 |
 八维超立方体 |
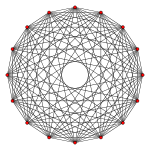 八维正轴体 |
九维
| 名称 | 施莱夫利 | 顶点 | 边 | 面 | 胞 | 4维胞 | 5维胞 | 维峰 | 维脊 | 维面 | χ |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 九维单体 | {38} | 10 | 45 | 120 | 210 | 252 | 210 | 120 | 45 | 10 | 2 |
| 九维超立方体 | {4,37} | 512 | 2304 | 4608 | 5376 | 4032 | 2016 | 672 | 144 | 18 | 2 |
| 九维正轴体 | {37,4} | 18 | 144 | 672 | 2016 | 4032 | 5376 | 4608 | 2304 | 512 | 2 |
 九维单体 |
 九维超立方体 |
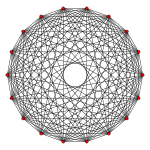 九维正轴体 |
十维
| 名称 | 施莱夫利 | 顶点 | 边 | 面 | 胞 | 4维胞 | 5维胞 | 6维胞 | 维峰 | 维脊 | 维面 | χ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 十维单体 | {39} | 11 | 55 | 165 | 330 | 462 | 462 | 330 | 165 | 55 | 11 | 0 |
| 十维超立方体 | {4,38} | 1024 | 5120 | 11520 | 15360 | 13440 | 8064 | 3360 | 960 | 180 | 20 | 0 |
| 十维正轴体 | {38,4} | 20 | 180 | 960 | 3360 | 8064 | 13440 | 15360 | 11520 | 5120 | 1024 | 0 |
 十维单体 |
 十维超立方体 |
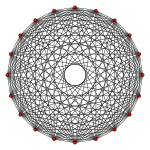 十维正轴体 |
十一维
| 名称 | 施莱夫利 | 顶点 | 边 | 面 | 胞 | 4维胞 | 5维胞 | 6维胞 | 7维胞 | 维峰 | 维脊 | 维面 | χ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 十一维单体 | {310} | 12 | 66 | 220 | 495 | 792 | 924 | 792 | 495 | 220 | 66 | 12 | 2 |
| 十一维超立方体 | {4,39} | 2048 | 11264 | 28160 | 42240 | 42240 | 29568 | 14784 | 5280 | 1320 | 220 | 22 | 2 |
| 十一维正轴体 | {39,4} | 22 | 220 | 1320 | 5280 | 14784 | 29568 | 42240 | 42240 | 28160 | 11264 | 2048 | 2 |
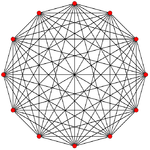 十一维单体 |
 十一维超立方体 |
 十一维正轴体 |
十二维
| 名称 | 施莱夫利 | 顶点 | 边 | 面 | 胞 | 4维胞 | 5维胞 | 6维胞 | 7维胞 | 8维胞 | 维峰 | 维脊 | 维面 | χ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 十二维单体 | {311} | 13 | 78 | 286 | 715 | 1287 | 1716 | 1716 | 1287 | 715 | 286 | 78 | 13 | 0 |
| 十二维超立方体 | {4,310} | 4096 | 24576 | 67584 | 112640 | 126720 | 101376 | 59136 | 25344 | 7920 | 1760 | 264 | 24 | 0 |
| 十二维正轴体 | {310,4} | 24 | 264 | 1760 | 7920 | 25344 | 59136 | 101376 | 126720 | 112640 | 67584 | 24576 | 4096 | 0 |
 十二维单体 |
 十二正轴体 |
更高维度
| 种类 | 维度 | 名称 | 施莱夫利 | 顶点 | 边 | 面 | 维峰 | 维脊 | 维面 | χ |
|---|---|---|---|---|---|---|---|---|---|---|
| 单纯形 | 13 | 十三维单体 | {312} | 14 | 91 | 364 | 364 | 91 | 14 | 2 |
| 14 | 十四维单体 | {313} | 15 | 105 | 455 | 455 | 105 | 15 | 0 | |
| 15 | 十五维单体 | {314} | 16 | 120 | 560 | 560 | 120 | 16 | 2 | |
| 16 | 十六维单体 | {315} | 17 | 136 | 680 | 680 | 136 | 17 | 0 | |
| 17 | 十七维单体 | {316} | 18 | 153 | 816 | 816 | 153 | 18 | 2 | |
| 18 | 十八维单体 | {317} | 19 | 171 | 969 | 969 | 171 | 19 | 0 | |
| 19 | 十九维单体 | {318} | 20 | 190 | 1140 | 1140 | 190 | 20 | 2 | |
| 20 | 二十维单体 | {319} | 21 | 210 | 1330 | 1330 | 210 | 21 | 0 | |
| 超方形 | 13 | 十三维超立方体 | {4,311} | 8192 | 53248 | 159744 | 2288 | 312 | 26 | 2 |
| 14 | 十四维超立方体 | {4,312} | 16384 | 114688 | 372736 | 2912 | 364 | 28 | 0 | |
| 15 | 十五维超立方体 | {4,313} | 32768 | 245760 | 860160 | 3640 | 420 | 30 | 2 | |
| 16 | 十六维超立方体 | {4,314} | 65536 | 524288 | 1966080 | 4480 | 480 | 32 | 0 | |
| 17 | 十七维超立方体 | {4,315} | 131072 | 1114112 | 4456448 | 5440 | 544 | 34 | 2 | |
| 18 | 十八维超立方体 | {4,316} | 262144 | 2359296 | 10027008 | 6528 | 612 | 36 | 0 | |
| 19 | 十九维超立方体 | {4,317} | 524288 | 4980736 | 22413312 | 7752 | 684 | 38 | 2 | |
| 20 | 二十维超立方体 | {4,318} | 1048576 | 10485760 | 49807360 | 9120 | 760 | 40 | 0 | |
| 正轴形 | 13 | 十三维正轴体 | {311,4} | 26 | 312 | 2288 | 159744 | 53248 | 8192 | 2 |
| 14 | 十四维正轴体 | {312,4} | 28 | 364 | 2912 | 372736 | 114688 | 16384 | 0 | |
| 15 | 十五维正轴体 | {313,4} | 30 | 420 | 3640 | 860160 | 245760 | 32768 | 2 | |
| 16 | 十六维正轴体 | {314,4} | 32 | 480 | 4480 | 1966080 | 524288 | 65536 | 0 | |
| 17 | 十七维正轴体 | {315,4} | 34 | 544 | 5440 | 4456448 | 1114112 | 131072 | 2 | |
| 18 | 十八维正轴体 | {316,4} | 36 | 612 | 6528 | 10027008 | 2359296 | 262144 | 0 | |
| 19 | 十九维正轴体 | {317,4} | 38 | 684 | 7752 | 22413312 | 4980736 | 524288 | 2 | |
| 20 | 二十维正轴体 | {318,4} | 40 | 760 | 9120 | 49807360 | 10485760 | 1048576 | 0 |
n维正非凸多胞形
从五维开始就都不存在任何非凸多胞形。
正无穷多胞形
一维
密铺
对应的欧几里得密铺只有一种,密铺于一维欧几里得空间,即直线,即正无限边形。其施莱夫利符号以{∞}表示、考克斯特符号以![]()
![]()
![]() 表示。
表示。
该镶嵌是由一维正图形“线段”(即二维二边形)完成一维欧几里得空间的密铺。
双曲密铺
对应的双曲密铺只有一种,即由一维正图形“线段”完成一维罗氏空间(即二维双曲线)的密铺,类似于无限边形,称为超无限边形,但又因为它是发散的,因此又称为伪多边形。在施莱夫利符号以{iπ/λ}表示、考克斯特符号以![]()
![]()
![]() 表示。
表示。
二维
平面正镶嵌图
| 名称 | 正方形镶嵌 | 正三角形镶嵌 | 正六边形镶嵌 |
|---|---|---|---|
| 对称群 | p4m, [4,4], (*442) | p6m, [6,3], (*632) | |
| 施莱夫利 {p,q} | {4,4} | {3,6} | {6,3} |
| 考克斯特记号 | |||
| 图像 | 
|

|

|
双曲凸正镶嵌图
| 双曲正镶嵌图 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 球面 (退化/柏拉图)/平面/双曲面 (庞加莱圆盘: 紧凑/仿紧凑/非紧凑) 镶嵌图与其施莱夫利符号 | |||||||||||
| p \ q | 2 | 3 | 4 | 5 | 6 | 7 | 8 | ... | ∞ | ... | iπ/λ |
| 2 |  {2,2} |
 {2,3} |
 {2,4} |
 {2,5} |
 {2,6} |
 {2,7} |
 {2,8} |
{2,∞} |
 {2,iπ/λ} | ||
| 3 |  {3,2} |
 (正四面体) {3,3} |
 (正八面体) {3,4} |
 (正二十面体) {3,5} |
 (三角镶嵌) {3,6} |
 {3,7} |
 {3,8} |
 {3,∞} |
 {3,iπ/λ} | ||
| 4 |  {4,2} |
 (立方体) {4,3} |
 (方形镶嵌) {4,4} |
 {4,5} |
 {4,6} |
 {4,7} |
 {4,8} |
 {4,∞} |
 {4,iπ/λ} | ||
| 5 |  {5,2} |
 (十二面体) {5,3} |
 {5,4} |
 {5,5} |
 {5,6} |
 {5,7} |
 {5,8} |
 {5,∞} |
 {5,iπ/λ} | ||
| 6 |  {6,2} |
 (六角镶嵌) {6,3} |
 {6,4} |
 {6,5} |
 {6,6} |
 {6,7} |
 {6,8} |
 {6,∞} |
 {6,iπ/λ} | ||
| 7 | {7,2} |
 {7,3} |
 {7,4} |
 {7,5} |
 {7,6} |
 {7,7} |
 {7,8} |
 {7,∞} |
{7,iπ/λ} | ||
| 8 | {8,2} |
 {8,3} |
 {8,4} |
 {8,5} |
 {8,6} |
 {8,7} |
 {8,8} |
 {8,∞} |
{8,iπ/λ} | ||
| ⋮ | |||||||||||
| ∞ |  {∞,2} |
 {∞,3} |
 {∞,4} |
 {∞,5} |
 {∞,6} |
 {∞,7} |
 {∞,8} |
 {∞,∞} |
 {∞,iπ/λ} | ||
| ⋮ | |||||||||||
| iπ/λ |  {iπ/λ,2} |
 {iπ/λ,3} |
 {iπ/λ,4} |
 {iπ/λ,5} |
 {iπ/λ,6} |
{iπ/λ,7} |
{iπ/λ,8} |
 {iπ/λ,∞} |
 {iπ/λ,iπ/λ} | ||
双曲星形正镶嵌图
| 名称 | 施莱夫利符号 | 考克斯特符号 | 图像 | 面的种类 {p} |
顶点图 {q} |
密度 | 对称 | 对偶 |
|---|---|---|---|---|---|---|---|---|
| 七阶七角星镶嵌 | {7/2,7} | 
|
{7/2} |
{7} |
3 | *732 [7,3] |
二分之七阶七边形镶嵌 | |
| 二分之七阶七边形镶嵌 | {7,7/2} | 
|
{7} |
{7/2} |
3 | *732 [7,3] |
七阶七角星镶嵌 | |
| 九阶九角星镶嵌 | {9/2,9} | 
|
{9/2} |
{9} |
3 | *932 [9,3] |
二分之九阶九边形镶嵌 | |
| 二分之九阶九边形镶嵌 | {9,9/2} | 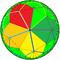
|
{9} |
{9/2} |
3 | *932 [9,3] |
九阶九角星镶嵌 | |
| 十一阶十一角星镶嵌 | {11/2,11} | 
|
{11/2} |
{11} |
3 | *11.3.2 [11,3] |
二分之十一阶十一边形镶嵌 | |
| 二分之十一阶十一边形镶嵌 | {11,11/2} | 
|
{11} |
{11/2} |
3 | *11.3.2 [11,3] |
十一阶十一角星镶嵌 | |
| p阶p角星镶嵌 | {p/2,p} | {p/2} | {p} | 3 | *p32 [p,3] |
二分之p阶p边形镶嵌 | ||
| 二分之p阶p边形镶嵌 | {p,p/2} | {p} | {p/2} | 3 | *p32 [p,3] |
p阶p角星镶嵌 | ||
| ... | ||||||||
| 无限阶无限角星镶嵌[注 11] | {∞/2,∞} | 
|
{∞/2} | {∞} | 3 | *∞.3.2 [∞,3] |
二分之无限阶无限边形镶嵌[注 11] | |
| 二分之无限阶无限边形镶嵌[注 11] | {∞,∞/2} | 
|
{∞} | {∞/2} | 3 | *∞.3.2 [∞,3] |
无限阶无限角星镶嵌[注 11] | |
三维
三维空间中只有一种正堆砌体,即立方体堆砌{4, 3, 4}:[7]
| 名称 | 施莱夫利 {p,q,r} |
考克斯特 |
胞 {p,q} |
面 {p} |
边图 {r} |
顶点图 {q,r} |
χ | 对偶 |
|---|---|---|---|---|---|---|---|---|
| 立方体堆砌 | {4,3,4} | {4,3} | {4} | {4} | {3,4} | 0 | 自身对偶 |
四维
| 名称 | 施莱夫利 {p,q,r,s} |
维面 {p,q,r} |
胞 {p,q} |
面 {p} |
面图 {s} |
边图 {r,s} |
顶点图 {q,r,s} |
对偶 |
|---|---|---|---|---|---|---|---|---|
| 超立方体堆砌 | {4,3,3,4} | {4,3,3} | {4,3} | {4} | {4} | {3,4} | {3,3,4} | 自身对偶 |
| 正十六胞体堆砌 | {3,3,4,3} | {3,3,4} | {3,3} | {3} | {3} | {4,3} | {3,4,3} | {3,4,3,3} |
| 正二十四胞体堆砌 | {3,4,3,3} | {3,4,3} | {3,4} | {3} | {3} | {3,3} | {4,3,3} | {3,3,4,3} |
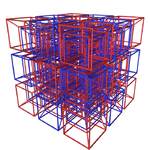 超立方体堆砌 |
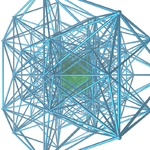 正十六胞体堆砌 |
 正二十四胞体堆砌 |
五维
五维空间的正堆砌仅有五维超立方体堆砌{4,3,3,3,4}[26]
| 名称 | 施莱夫利 {p,q,r,s,t} |
考克斯特 |
维面 {p,q,r,s} |
维脊 {p,q,r} |
胞 {p,q} |
面 {p} |
边图 {t} |
顶点图 {s,t} |
对偶 |
|---|---|---|---|---|---|---|---|---|---|
| 五维超立方体堆砌 | {4,3,3,3,4} | {4,3,3,3} | {4,3,3} | {4,3} | {4} | {4} | {3,4} | 自身对偶 |
六维以上
| δn | 维度 | 名称 | 施莱夫利 | 考克斯特 | |||
|---|---|---|---|---|---|---|---|
| 原位 {∞}n (2m色, m<n) |
正 {4,3n-1,4} (1色、n色) |
网格 {4,3n-4,31,1} (2色) | |||||
| δ6 | 五维(退化六维) | 五维超立方体堆砌 | {∞}5 {4,33,4} {4,32,31,1} |
||||
| δ7 | 六维(退化七维) | 六维超立方体堆砌 | {∞}6 {4,34,4} {4,33,31,1} |
||||
| δ8 | 七维(退化八维) | 七维超立方体堆砌 | {∞}7 {4,35,4} {4,34,31,1} |
||||
| δ9 | 八维(退化九维) | 八维超立方体堆砌 | {∞}8 {4,36,4} {4,35,31,1} |
||||
| δn | n-1维(退化n维) | n-1维超立方体堆砌 | {∞}n {4,3n-3,4} {4,3n-4,31,1} |
... | |||
双曲
六维或以上的维度皆不存在紧空间与仿紧空间的双曲堆砌。不过,任何的{p,q,r,s,...}形式(其中p,q,r,s,...是大于二的自然数或无限大)以上并不包括n维空间的非紧镶嵌。
非紧镶嵌
| 维 | 总数 | 群 | ||||
|---|---|---|---|---|---|---|
| 三维(退化四维) | ∞ | [3,3,7] ... [∞,∞,∞]: [4,3[3]] ... [∞,∞[3]]: | ||||
| 四维(退化五维) | 186 | ...[3[3,3,3]]: |
||||
| 五维(退化六维) | 66 | |||||
| 六维(退化七维) | 36 | [31,1,1,1,1,1]: | ||||
| 七维(退化八维) | 13 |
[3,3,3[6]]: |
[4,3,3,33,1]: |
[4,3,3,32,2]: | ||
| 八维(退化九维) | 10 |
[3,3[3+4],3]: |
[32,1,32,32,1]: |
[33,1,33,4]: [33,1,3,3,31,1]: |
[33,3,2]: [32,2,4]: | |
| 九维(退化十维) | 8 | [3,3[8],3]: [3,3[3+5],3]: |
[32,1,33,32,1]: |
[35,3,1]: [33,1,34,4]: |
[34,4,1]: | |
| 十维(退化十一维) | 4 | [32,1,34,32,1]: |
[32,1,36,4]: [32,1,35,31,1]: |
[37,2,1]: | ||
复合正图形
二维复合正多边形
 2{2} |
3{2} |
 4{2} |
5{2} |
 6{2} |
7{2} |
 8{2} |
9{2} |
 10{2} |
 11{2} |
 12{2} |
 13{2} |
 14{2} |
 15{2} | |
2{3} |
 3{3} |
 4{3} |
 5{3} |
6{3} |
 7{3} |
 8{3} |
 9{3} |
 10{3} |
 2{4} |
 3{4} |
 4{4} |
 5{4} |
 6{4} |
 7{4} |
2{5} |
 3{5} |
 4{5} |
 5{5} |
 6{5} |
2{5/2} |
 3{5/2} |
 4{5/2} |
 5{5/2} |
 6{5/2} |
 2{6} |
3{6} |
 4{6} |
 5{6} | |
2{7} |
 3{7} |
 4{7} |
2{7/2} |
 3{7/2} |
 4{7/2} |
2{7/3} |
 3{7/3} |
 4{7/3} |
 2{8} |
 3{8} |
 2{8/3} |
 3{8/3} | ||
2{9} |
 3{9} |
2{9/2} |
 3{9/2} |
2{9/4} |
 3{9/4} |
 2{10} |
 3{10} |
 2{10/3} |
 3{10/3} | |||||
 2{11} |
 2{11/2} |
 2{11/3} |
 2{11/4} |
 2{11/5} |
 2{12} |
 2{12/5} |
 2{13} |
 2{13/2} |
 2{13/3} |
 2{13/4} |
 2{13/5} |
 2{13/6} | ||
 2{14} |
 2{14/3} |
 2{14/5} |
 2{15} |
 2{15/2} |
 2{15/4} |
 2{15/7} |
抽象正图形
多面体 
内侧菱形三十面体
十二合十二面体
内侧三角星化二十面体
双三斜十二面体
凹五角锥十二面体顶点图 {5}, {5/2} 

(5.5/2)2 
{5}, {5/2} 

(5.5/3)3 
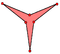
面 30个菱形 
12个五边形
12个五角星

20个六边形 
12个五边形
12个五角星

20个六边形 
镶嵌 
{4, 5}
{5, 4}
{6, 5}
{5, 6}
{6, 6}χ −6 −6 −16 −16 −20
参见
注释
参考文献
- Coxeter, The Beauty of Geometry: Twelve Essays, Dover Publications, 1999 互联网档案馆) PDF
- D. M. Y. Sommerville, An Introduction to the Geometry of n Dimensions. New York, E. P. Dutton, 1930. 196 pp. (Dover Publications edition, 1958) Chapter X: The Regular Polytopes
- ^ 1.0 1.1 1.2 1.3 1.4 1.5 1.6 George Maxwell, Sphere Packings and Hyperbolic Reflection Groups Archive.is的存档,存档日期2013-06-30, JOURNAL OF ALGEBRA 79,78-97 (1982)
- ^ Hao Chen, Jean-Philippe Labbé, Lorentzian Coxeter groups and Boyd-Maxwell ball packings, http://arxiv.org/abs/1310.8608 (页面存档备份,存于互联网档案馆)
- ^ Wolcott, Luke; McTernan, Elizabeth. Imagining Negative-Dimensional Space (PDF). Bosch, Robert; McKenna, Douglas; Sarhangi, Reza (编). Proceedings of Bridges 2012: Mathematics, Music, Art, Architecture, Culture. Phoenix, Arizona, USA: Tessellations Publishing: 637–642. 2012 [10 July 2015]. ISBN 978-1-938664-00-7. ISSN 1099-6702. (原始内容 (PDF)存档于2015-06-26).
- ^ 4.0 4.1 McMullen, Peter; Schulte, Egon, Abstract Regular Polytopes 1st, Cambridge University Press, December 2002, ISBN 0-521-81496-0
- ^ zero dimensional. planetmath.org. [2015-06-06]. (原始内容存档于2015-06-24).
- ^ Hazewinkel, Michiel. Encyclopaedia of Mathematics, Volume 3. Kluwer Academic Publishers. 1989: 190 [2016-07-15]. (原始内容存档于2019-09-05).
- ^ 7.0 7.1 7.2 7.3 7.4 7.5 7.6 Coxeter, Regular Polytopes, 3rd. ed., Dover Publications, 1973. ISBN 0-486-61480-8. (Tables I and II: Regular polytopes and honeycombs, pp. 294–296)
- ^ Coxeter Regular Polytopes(1973)[7], p. 129
- ^ Abstract Regular Polytopes(2002)[4], p. 30
- ^ Johnson (2012), p. 86
- ^ Coxeter Regular Polytopes(1973)[7], p. 120
- ^ Coxeter Regular Polytopes(1973)[7], p. 124
- ^ Richelot, Friedrich Julius. De resolutione algebraica aequationis X257 = 1, sive de divisione circuli per bisectionem anguli septies repetitam in partes 257 inter se aequales commentatio coronata. Journal für die reine und angewandte Mathematik. 1832, 9: pp. 1–26, 146–161, 209–230, 337–358 (拉丁语).
- ^ Coxeter, H. S. M. Introduction to Geometry 2nd ed. New York: Wiley. February 1989. ISBN 978-0-471-50458-0.
- ^ Hermes, Johann Gustav. Ueber die Teilung des Kreises in 65537 gleiche Teile. Nachrichten von der Gesellschaft der Wissenschaften zu Göttingen, Mathematisch-Physikalische Klasse (Göttingen). 1894, 3: pp. 170–186 (德语).
- ^ Hermes, Johann Gustav. Ueber die Teilung des Kreises in 65537 gleiche Teile. Nachrichten von der Gesellschaft der Wissenschaften zu Göttingen, Mathematisch-Physikalische Klasse (Göttingen). 1894, 3: pp. 170–186 [2016-08-10]. (原始内容存档于2017-01-11) (德语).
- ^ Weisstein, Eric W. (编). 257-gon. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Weisstein, Eric W. (编). 65537-gon. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Darling, David J., The universal book of mathematics: from Abracadabra to Zeno's paradoxes, John Wiley & Sons, 2004. Page 249. ISBN 0-471-27047-4.
- ^ Russell, Bertrand, History of Western Philosophy (页面存档备份,存于互联网档案馆), reprint edition, Routledge, 2004, p. 202, ISBN 0-415-32505-6.
- ^ Coxeter, Introduction to geometry, 1969, Second edition, sec 21.3 Regular maps, p. 386-388
- ^ Weisstein, Eric W. (编). Digon. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Weisstein, Eric W. (编). Pentagram. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Regular Skew Polyhedra in three and four dimensions and their topological analogues, Proceedings of the London Mathematics Society, Ser. 2, Vol 43, 1937.
- ^ Coxeter Regular Polytopes(1973)[7], Table I: Regular polytopes, (iii) The three regular polytopes in n dimensions (n>=5), pp. 294–295.
- ^ Coxeter Regular Polytopes(1973)[7], p. 296, Table II: Regular honeycombs
外部链接
- The Platonic Solids (页面存档备份,存于互联网档案馆)
- Kepler-Poinsot Polyhedra (页面存档备份,存于互联网档案馆)
- Regular 4d Polytope Foldouts
- Multidimensional Glossary (Look up Hexacosichoron and Hecatonicosachoron)
- Polytope Viewer (页面存档备份,存于互联网档案馆)
- Polytopes and optimal packing of p points in n dimensional spheres
- An atlas of small regular polytopes (页面存档备份,存于互联网档案馆)
| ||||||||||||||||||||||